The differentiation of e^cotx is equal to -cosec2x ecotx. In this post, we will learn how to differentiate e to the power cotx with respect to x.
The following two formulas will be used to find the derivative of ecotx.
- d/dx(cotx) = -cosec2x.
- loga ak =k.
Now, we will learn to find the derivative of e to the power cotx with respect to x.
How to Find the derivative of ecotx
Question: How to differentiate ecotx?
Solution:
Let y= ecotx
Taking natural logarithms on both sides, we get that (natural logarithm means the logarithm with base e, i.e. loge)
log y = cotx
[Here we have used the above formula 2]
Now, differentiate both sides with respect to x. This will give us
$\dfrac{1}{y} \dfrac{dy}{dx}$ = -cosec2x, by the above formula 1.
⇒ $\dfrac{dy}{dx}$ = -y cosec2x
Now put back the value of y, that is, y=ecotx. Therefore, we obtain that
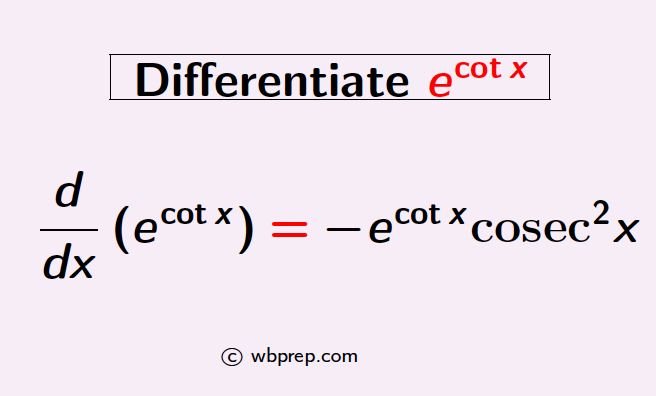
$\dfrac{d}{dx}$(ecotx) = – ecotx cosec2x.
Thus, the differentiation of ecotx with respect to x is equal to -ecotx cosec2x.
ALSO READ:
Derivative of arc(cotx): The derivative of arc(cotx) is -1/(1+x2).
Differentiate esinx: The derivative of esinx is esinx cosx.
Differentiate ecosx: The derivative of ecosx is -ecosx sinx.
Differentiate etanx: The derivative of etanx is etanx sec2x.
FAQs
Q1: What is the derivative of ecotx?
Answer: The derivative of e^cotx is equal to -cosec2x ecotx.
Q2: If y=ecotx, then find dy/dx?
Answer: If y=ecotx, then dy/dx = -cosec2x ecotx.