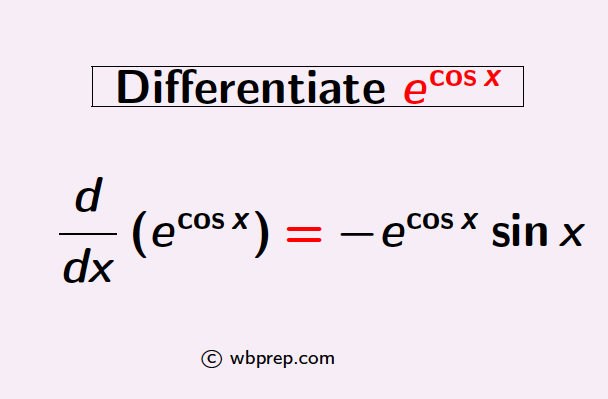
The differentiation of e^cosx is equal to -sinx ecosx. In this post, we will learn how to differentiate e^cosx with respect to x.
Let us find the derivative of ecosx with respect to x.
Find the derivative of ecosx
Question: How to differentiate ecosx?
Solution:
Let y= ecosx
Taking natural logarithms on both sides, we get that
logy = cosx [here we use the logarithm formula logeem =m]
Differentiating both sides with respect to x, we get that
$\dfrac{1}{y} \dfrac{dy}{dx}$ = -sinx
⇒ $\dfrac{dy}{dx}$ = -y sinx
Now we put the value of y, that is, y=ecosx. By doing so we obtain that
$\dfrac{d}{dx}$(ecosx) = -ecosx sinx.
Thus, the differentiation of ecosx with respect to x is equal to -ecosx sinx.
Video solution:
ALSO READ:
Derivative of arc(cotx): The derivative of arc(cotx) is -1/(1+x2).
Derivative of esinx: The derivative of esinx is cosx esinx.
FAQs
Q1: What is the derivative of ecosx?
Answer: The derivative of e^cosx is equal to -sinx ecosx.
Q2: If y=ecosx, then find dy/dx?
Answer: If y=ecosx, then dy/dx = -sinx ecosx.