How to Solve Linear Equations

A linear equation in one variable is always of the form ax+b=c, where a, b, and c are constants. Linear equations are also known as simple equations. In this post, we will learn how to solve linear equations with examples.

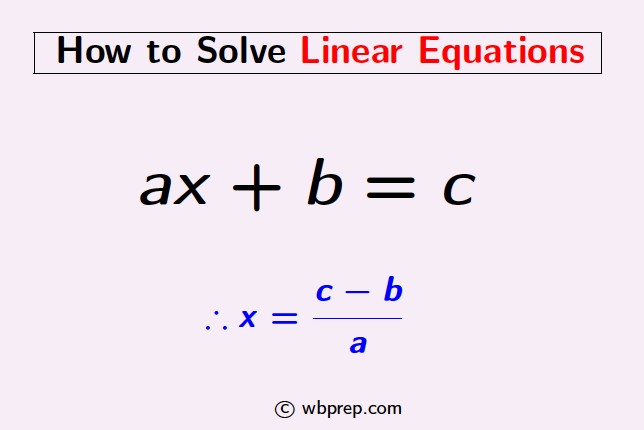
ax+b=c

Put c=0.

Then ax+b = 0

⇒ ax = -b

This is an equation of a straight line passing through the origin (0, 0).

How to Solve ax+b=c

We will follow the below steps to solve a linear equation of the form ax+b=c.

Step 1	First, we will separate the variable x and the constant terms.
Step 2	To do so, let us bring +b into the right-hand side. So it will be negative on the other side. Hence, ax = c-b …(∗)
Step 3	Divide both sides of (∗) by the coefficient of x which is a.
Step 4	⇒ ax/a = (c-b)/a
Step 5	Canceling a in the left-hand side, we get that x=(c-b)/a
Step 6	Simplify the fraction (c-b)/a if necessary, the resulting quantity will be the solution of the given equation ax+b =c.

Let us now understand the above method of solving a linear equation with examples. We will start with the example below.

Example 1:

Solve 6x=18

Answer:

Given 6x=18

See that the variable and the constant term are already separated. Thus, in order to get the value of x, we will divide both sides by the coefficient of x which is 6.

Dividing both sides of 6x=18 by 6, we get that

$\dfrac{6x}{6}=\dfrac{18}{6}$

⇒ $\dfrac{\cancel{6}x}{\cancel{6}}=\dfrac{\cancel{6} \times 3}{\cancel{6}}$

⇒ x=3.

So x=3 is the solution of the linear equation 6x=18.

Video Solution of 6x=18:

ALSO READ:

How to solve x⁴+x²+1=0

Solve x²+25=0

FAQs